Creating a variational formulation in the Unified Form Language (UFL)#
We have previously seen how to define a finite element, and evaluate its basis functions in points on the reference element. However, in this course we aim to solve problems from solid mechanics. Thus, we need more than the basis functions to efficiently solve the problems at hand.
In this section, we will introduce the Unified Form Language UFL, which is a domain-specific language for defining variational formulations for partial differential equations. The goal of this section is to be able to solve the following problem:
Given to function \(f\) and \(g\), compute the approximation of \(\frac{f}{g}\) in a specified finite element space. Mathematically, we can write
Find \(u\in V(\Omega)\) such that
We start by focusing on the computational domain \(\Omega\),
The computational domain#
The general idea of the finite element method is to sub-divide \(\Omega\) into smaller (polygonal) elements \(K_j\) such that
The triangulation covers \(\Omega\): \(\cup_{j=1}^{M}K_j=\bar{\Omega}\)
No overlapping polyons: \(\mathrm{int} K_i \cap \mathrm{int} K_j=\emptyset\) for \(i\neq j\).
No vertex lines in the interior of a facet or edge of another element
We will call our polygonal domain \(\mathcal{K}={K_j}_{j=1}^{M}\). Next, we define a reference element \(K_{ref}\), which is a simple polygon that we can map to any element \(K_j\), using the mapping \(F_j:K_{ref}\mapsto K_j\).
We define the Jacobian of this mapping as \(\mathbf{J_j}\).
Example: Straight edged triangle#
As we saw in the section on finite elements, we can use basix to get a sample of points within the reference element.
import basix.ufl
import numpy as np
reference_points = basix.create_lattice(basix.CellType.triangle, 13,
basix.LatticeType.gll, exterior=False,
method=basix.LatticeSimplexMethod.warp)
Next, we realize that we can use the first order Lagrange space, to represent the mapping from the reference element to any physical element: Given three points, \(p_0=(x_0, y_0)^T, p_1=(x_1,y_1)^T, p_2=(x_2,y_2)^T\), we can represent any point \(x\) as the linear combination of the three basis functions on the reference element \(X\).
def compute_physical_point(p0, p1, p2, X):
"""
Map coordinates `X` in reference element to triangle defined by `p0`, `p1` and `p2`
"""
el = basix.ufl.element("Lagrange", "triangle", 1)
basis_values = el.tabulate(0, X)
return (basis_values[0] @ np.vstack([p0, p1, p2]))
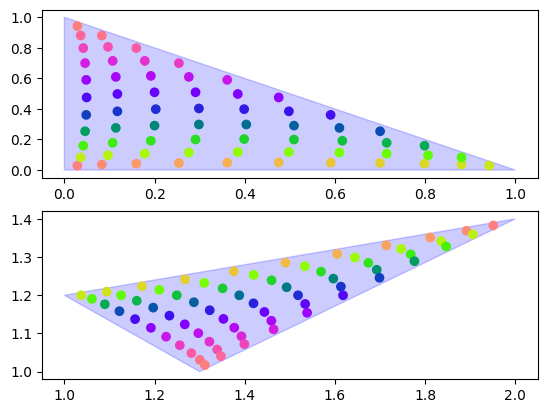
We can now experiment with this code
p0 = np.array([2.0, 1.4])
p1 = np.array([1.0, 1.2])
p2 = np.array([1.3, 1.0])
x = compute_physical_point(p0, p1, p2, reference_points)
We use matplotlib to visualize the reference points and the physical points
import matplotlib.pyplot as plt
theta = 2 * np.pi
phi = np.linspace(0, theta, reference_points.shape[0])
rgb_cycle = (np.stack((np.cos(phi),
np.cos(phi-theta/4),
np.cos(phi+theta/4)
)).T
+ 1)*0.5 # Create a unique colors for each node
fig, (ax_ref, ax) = plt.subplots(2, 1)
# Plot reference points
reference_vertices = basix.cell.geometry(basix.CellType.triangle)
ref_triangle= plt.Polygon(reference_vertices, color="blue", alpha=0.2)
ax_ref.add_patch(ref_triangle)
ax_ref.scatter(reference_points[:,0], reference_points[:,1], c=rgb_cycle)
# Plot physical points
vertices = np.vstack([p0, p1, p2])
triangle = plt.Polygon(vertices, color="blue", alpha=0.2)
ax.add_patch(triangle)
ax.scatter(x[:,0], x[:,1], c=rgb_cycle)
<matplotlib.collections.PathCollection at 0x7f7744d52d70>
Exercises:#
Can we use a similar kind of mapping on a quadrilateral/tetrahedral/hexahedral element?
What happens if we change the order of the basis functions?
How can we compute the Jacobian of the mapping?
Using UFL to define a symbolic representation of a domain#
As seen above, we can use the Lagrange element basis functions to represent the mapping from the reference element to the physical element (and its inverse).
In the unified form language we make a symbolic representation of the computational domain, using ufl.Mesh.
import basix.ufl
import ufl
cell = "triangle"
c_el = basix.ufl.element("Lagrange", cell, 1, shape=(2,))
Note that if we wanted to represent a 2D manifold represented in 3D, we could change the shape-parameter to (3, ).
We call this element the coordinate element, as it represents the transformation of coordinates between the reference
element and the physical element.
domain = ufl.Mesh(c_el)
We note that this is a purely symbolic representation, it doesn’t matter if we are solving something on a unit square, on a 2D representation of a bridge or a brain. The only thing that matters is what kind of elements we use to represent the domain.
The function space#
Once we have subdivided \(\Omega\) into elements \(K\), we can define a discrete function space \(V_h\):
Certain finite elements need to conserve certain properties (0-valued normals components of facets, etc). We define this map as: \((\mathcal{F}_j(\phi))(x)\).
For the finite elements we will consider in this tutorial, we will use the map
where \(\hat\phi\) is the basis function on the reference element. In other words, to evaluate the basis function in a point in the physical space, we pull the point back to the reference element evaluate our local basis function at this point
Thus, we can write out the evaluation of a finite element function as
As opposed some commercial software, we do not rely on iso-parametric elements in FEniCS.
We can use any supported finite element space to describe our unknown u.
For more advanced maps, see for instance: DefElement - vector valued basis functions.
el = basix.ufl.element("Discontinuous Lagrange", cell, 2)
V = ufl.FunctionSpace(domain, el)
For the coefficients f and g, we choose
F = ufl.FunctionSpace(domain, basix.ufl.element("Discontinuous Lagrange", cell, 0))
f = ufl.Coefficient(F)
G = ufl.FunctionSpace(domain, basix.ufl.element("Lagrange", cell, 1))
g = ufl.Coefficient(G)
The variational form of a projection#
The finite element method and its links to minimization
Recall that the variational form is a way of writing the PDE on a form that we can use to solve the problem with FEM. We start by multiplying the equation with a test-function from a suitable space (in this case the same space as the solution) and integrate over the domain.
This is also equivalent to minimizing the following functional
We find the minimum by computing \(\frac{\mathrm{d}{J}}{\mathrm{d}u}[\delta u]=0\).
We obtain them in the standard way, by multiplying by a test function
and integrating over the domain, we do this by using dx, which means that we integrate over all cells of the mesh.
u = ufl.TrialFunction(V)
v = ufl.TestFunction(V)
a = ufl.inner(u, v) * ufl.dx
Note that the bi-linear form a is defined with trial and test functions.
L = ufl.inner(f / g, v) * ufl.dx
forms = [a, L]
So far, so good?
As opposed to most demos/tutorials on FEniCSx, note that we have not imported dolfinx
or made a reference to the actual computational domain we want to solve the problem on or what f or g is,
except for the choice of function spaces.
Further analysis of the variational form#
We next use the map \(F_K:K_{ref}\mapsto K\) to map the integrals over each cell in the domain back to the reference cell.
where \(K\) is each element in the physical space, \(J_K\) the Jacobian of the mapping.
Next, we can insert the expansion of \(u, v, f, g\) into the formulation: \(u=\sum_{i=0}^{\mathcal{N}}u_i\phi_i(x)\qquad v=\sum_{i=0}^{\mathcal{N}}v_i\phi_i(x)\qquad f=\sum_{k=0}^{\mathcal{M}}f_k\psi_k(x)\qquad g=\sum_{l=0}^{\mathcal{T}}g_l\varphi_l(x)\)
and identify the matrix system \(Au=b\), where
Warning
Next, one can choose an appropriate quadrature rule with points and weights, include the correct mapping/restrictions of degrees of freedom for each cell. All of this becomes quite tedious and error prone work, and has to be repeated for every variational form!
Since this is time consuming work, we will use UFL to compute these operations for us. Below is an example of how we can use UFL on the variational form above to apply the pull back to the reference element.
pulled_back_L = ufl.algorithms.compute_form_data(
L,
do_apply_function_pullbacks=True,
do_apply_integral_scaling=True,
do_apply_geometry_lowering=True,
preserve_geometry_types=(ufl.classes.Jacobian,),
)
print(pulled_back_L.integral_data[0])
IntegralData over domain(cell, ('otherwise',)) with integrals:
{ weight * |(J[0, 0] * J[1, 1] + -1 * J[0, 1] * J[1, 0])| * (reference_value(w_0)) / (reference_value(w_1)) * (reference_value(v_0)) } * dx(<Mesh #0>[('otherwise',)], {'estimated_polynomial_degree': 3})
and metadata:
{metadata}
Functionals and derivatives#
As mentioned above, many finite element problems can be rephrased as an optimization problem.
For instance, we can write the equations of linear elasicity as an optimization problem:
where \(C\) is the stiffness tensor given as \(C_{ijkl} = \lambda \delta_{ij}\delta_{kl} + \mu(\delta_{ik}\delta_{jl}+\delta_{il}\delta{kj})\), \(\epsilon\) is the symmetric strain tensor and \(u_h\) a displacement field.
We start by defining these quantities in UFL:
The function space for displacement
el = basix.ufl.element("Lagrange", cell, 1, shape=(2,))
Vh = ufl.FunctionSpace(domain, el)
uh = ufl.Coefficient(Vh)
f = ufl.Coefficient(Vh)
Lame’s elasticity parameters
mu = ufl.Constant(domain)
lmbda = ufl.Constant(domain)
def epsilon(u):
return ufl.sym(ufl.grad(u))
We define the stiffness tensor using Einstein summation notation. We start by defining the identity tensor which we will use as a Kronecker Delta function. Next we define four indices that we will use to account for the four dimensions of the stiffness tensor.
Id = ufl.Identity(domain.geometric_dimension())
indices = ufl.indices(4)
Secondly we define the product of two delta functions \(\delta_{ij}\delta_{kl}\) which results in a fourth order tensor.
def delta_product(i, j, k, l):
return ufl.as_tensor(Id[i, j] * Id[k, l], indices)
# Finally we define the Stiffness tensor
i,j,k,l = indices
C = lmbda * delta_product(i,j,k,l) + mu*(delta_product(i,k,j,l) + delta_product(i,l,k,j))
and the functional
Jh = 0.5*(C[i,j,k,l] * epsilon(uh)[k,l]) * epsilon(uh)[i,j] * ufl.dx - ufl.inner(f, uh) * ufl.dx
This syntax is remarkably similar to how it is written on paper.
Alternative formulation#
Instead of writing out all the indices with Einstein notation, one could write the same equation as
def sigma(u):
return lmbda * ufl.nabla_div(u) * ufl.Identity(len(u)) + 2 * mu * epsilon(u)
Jh = 0.5 * ufl.inner(sigma(uh), epsilon(uh)) * ufl.dx - ufl.inner(f, uh) * ufl.dx
Differentiating the energy functional#
We can differentiate the energy functional with respect to the displacement field \(u_h\).
F = ufl.derivative(Jh, uh)
Since we want to find the minimum of the functional, we set the derivative to zero. To solve this problem, we can for instance use a Newton method, where we solve a sequence of equations:
where \(J_F\) is the Jacobian matrix of \(F\). We can rewrite this as:
Which boils down to solving a linear system of equations for \(\delta u_k\).
We can compute the Jacobian using UFL:
J_F = ufl.derivative(F, uh)
And with this and \(F\) we can solve the minimization problem. See for instance: Custom Newton Solver in DOLFINx for more details about how you could implement this method by hand.
Extra material: Optimization problems with PDE constraints#
Another class of optimization problems is the so-called PDE-constrained optimization problems. For these problems, one usually have a problem on the form
such that
We can use the adjoint method to compute the sensitivity of the functional with respect to the solution of the PDE. This is done by introducing the Lagrangian
We now seek the minimum of the Lagrangian, i.e.
which we can write as
Since \(\lambda\) is arbitrary, we choose \(\lambda\) such that
for any \(\delta u\) (including \(\frac{\mathrm{d}u}{\mathrm{d}c}[\delta c]\)).
This would mean that
To find such a \(\lambda\), we can solve the adjoint problem
With UFL, we do not need to derive these derivatives by hand, and can use symbolic differentiation to get the left and right hand side of the adjoint problem.
dFdu_adj = ufl.adjoint(ufl.derivative(F, uh))
dJdu_adj = ufl.derivative(Jh, uh)
dJdf = ufl.derivative(Jh, f)
dFdf = ufl.derivative(F, f)